Applications of Mathematics in Finance

What is mathematical finance?

Financial mathematics is the application of mathematical methods within the financial field. It utilises ideas from statistics, probability, and economic theory to find optimised solutions to financial problems.

Financial mathematics has started to incorporate much more complex mathematics to solve more complex problems in recent years and it is only growing, especially with the development of quantitative analysis and trading.

There are many branches of mathematics used in finance, but some interesting ideas come from game theory such as the differential game method and statistical modelling leading to ideas like the CAPM and the Monte Carlo method, which are outlined below.

Differential Game Method

Differential games are a group of problems related to the analysis of conflict in the context of a dynamical system and is studied within game theory.

They are closely related to optimal control problems. In optimal control problems there is single control  a function of time, and a single criterion to be optimized; differential game theory generalizes this to two controls and two criteria, one for each player.

Players attempt to control the state of the system to achieve their goal and the system responds to the inputs of all players. An example of an optimal control problem would be solving for the shadow price (costate) .

It works by giving a quantifiable value to reducing or increasing the state variable in the next turn. It can sometimes be solved analytically, but mostly there would be a margin of solutions however by reducing the problem into a dynamical system we can solve for an exact value. 

When financial markets do not obey the steady state hypothesis and fluctuations occur, typically the change in fluctuations is also abnormal so we need to use a stochastic dynamic model to analyse security investments and by using the differential game method we can accommodate these fluctuations to get the most optimal solution.

RELATED Bain Capital acquires majority stake in Adani Capital and Adani Housing Finance

Capital Asset Pricing Model

The capital asset pricing model (CAPM) is one of the most widely known uses of mathematics in finance. It is a model that calculates the expected rate of return of an asset.

It uses the return on both the market and a risk-free asset as well as a number that quantifies how sensitive the asset (the one which we are trying to calculate the return for) is to changes in the market (we call this value the beta) to calculate the return.

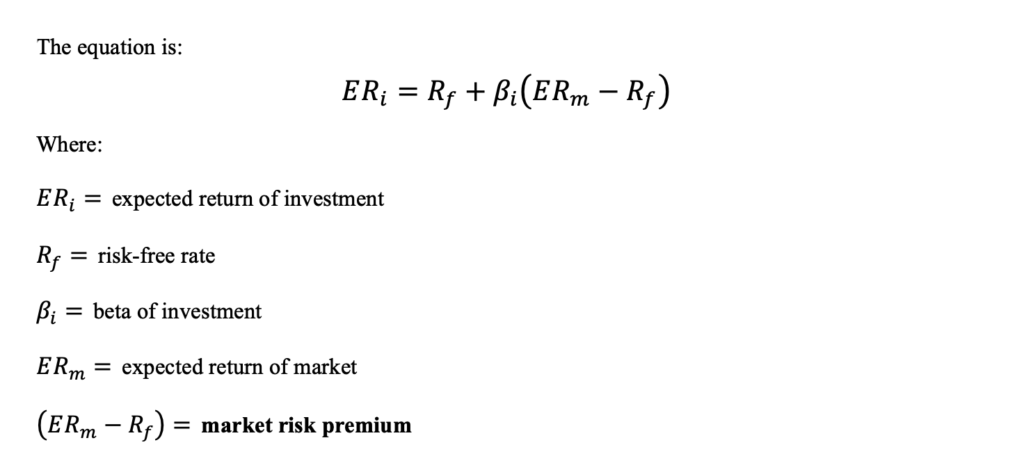
There are downsides to this model that lie on the assumptions made based on modern financial theory that may not hold up in the real world.

For one it assumes security markets are efficient and competitive, and secondly it assumes that these markets are predominantly made up by rational, risk-averse investors, who want to maximize returns on their investments.

RELATED Apollo Global Management rescues Carvana with a restructuring deal

Monte Carlo Simulations

When analysts typically look at portfolios and want to gage the best returns, they would look at all historic data to see what has occurred.

This method can be improved upon by using the Monte Carlo simulation, which allows us to obtain a distribution of results for any statistical problem with multiple inputs sampled repeatedly.

 A way to understand the method is to consider a gambler with no knowledge of the probability of dice throws and they are playing a game to get a six in total for any two consecutive throws. Now we would like to consider the possibility of them rolling a three and three.

What would be the probability of this, especially if we had an unfair die? We would roll the dice multiple times ideally millions of times and count the number of consecutive threes we get to find an estimation of the probability.

This idea can be simulated with the Monte Carlo method much more efficiently. Whilst asset prices do not typically behave like a die, they can be seen as random walks, which can be simulated showing a distribution of results with probabilistic outcomes, so to not rely solely on historical data. 

In conclusion financial mathematics is only going to grow in its influence on the investment world as it’s utility increases across all fields.

Glossary:

Dynamical system: A system whose state evolves with time over a state space (a set of all possible states) according to fixed rules.

Shadow price: The estimated price of something that is not typically sold in the market or not normally priced.

Steady state hypothesis: When all sources of competitive advantage are exhausted and therefore the profitability and efficiency rates stabilise.

Stochastic dynamic model: A method to predict statistical properties of a set of outcomes by considering random variance in any parameters used.

Market risk premium: The rate of return on a risky investment.

Random walks: A random path that is a succession of random steps in a mathematical space.

To get in contact with feedback on this article please email us at publishing@krugmaninsights.com